The contrast transfer function (MTF) – The central element for determining resolving power

You are here: Nature Science Photography – Visual acuity – Image sharpness II

We specify the resolving power of our imaging and output devices in line pairs per millimeter (Lp/mm). At first glance, this purely technical measure seems strange because our subjects consist of more or less complicated structures, smooth or abrupt tonal value transitions, and coarse or fine object details. But if we break it down, we can think of each object as a sum of periodic structures of varying fineness and orientation. The resolution test with the bar grid is just a very simple example of this. Instead of abrupt transitions from black to white, in reality, we just have to imagine soft transitions. They form the object structure that we image with our imaging system. On the way through, the contrast is weakened according to the contrast transfer function (MTF) of each component, so that the resulting image is only a more or less good equivalent of the object.

The high spatial frequencies of the MTF correspond to fine image details. The more extended the curve, the finer the details are reproduced and the sharper the image appears.

We used the bar test for an extended period to determine how many line pairs a system component can resolve. For example, the optics to be tested were used to expose the test specimen to a film with the highest resolution possible. Then, using a magnifying glass or microscope to look at the film, it was decided how many line pairs per unit length (usually per millimeter) could still be seen. Since human perception and judgment were involved in this method of determining resolution, inconsistent results were inevitable. The number of line pairs per millimeter would have been more meaningful if its determination had been made at a fixed contrast level. However, because of the instrumentation required, this was very difficult to meet.

The introduction of the contrast transfer function at Carl Zeiss in Jena in the 1940s solved the problem of determining resolution while taking perceived sharpness into account. The MTF tests the contrast of a spatial frequency f relative to the lowest frequency, which is 100%, thereby assuming that the original contrast remains constant across all frequencies. Let’s examine the mathematical components before defining the function:

VS - The minimum brightness of a black area at the lowest spatial frequency
VW - The maximum brightness of a white area at the lowest spatial frequency
Vmin - The minimum brightness of the test pattern at the spatial frequency under test f 
Vmax - The maximum brightness of the test pattern at the spatial frequency under test f 
C (0) - ((VW-VS)/(VW+VS)) gives the contrast at the lowest spatial frequency
C (f) - ((Vmax-VMin)/(VMax+VMin)) gives the contrast at the spatial frequency f under test. The division by the term (VMax+VMin) reduces possible errors in the acquisition of the test pattern

The contrast transfer function for a spatial frequency f to be tested is then defined as follows:

Formula 28

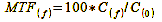
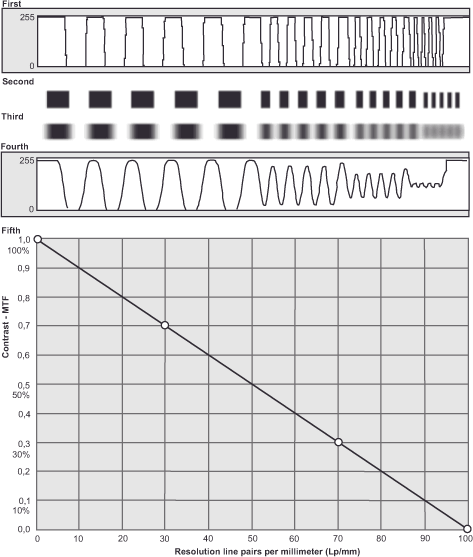
That is rather theoretical, and one can imagine little under it, I know. The process becomes clearer if we relate the MTF to something tangible, as figure 39
(Derivation of the Modulation Transfer Function)

does. In 1. we see a test pattern of dark and light stripes (bar test). There are four sets of stripes, each closer together. In 2. we see a profile of this test pattern, i.e., a representation of the brightness of the test pattern from 1. As a concession to the unstoppable digitization, we denote the highest brightness of the white areas with 255 and the lowest of the blacks with 0, because 0 to 255 is the brightness range in a digital image coded with 8 bits. 3. This reflects the potential appearance of the test pattern when viewed through a lens. The black and white areas are blurred, and this blurring increases the closer together they are. 4. then shows the brightness profile of this hypothetical image with the MTF values that result. 5. puts this into another form. There, we present the MTF value as a percentage of the contrast on the y-axis, and the number of line pairs per millimeter on the x-axis. From the transfer of the brightness distribution into the MTF diagram, it can be seen that the image contrast of the coarse structures on the far left is equal to 1, i.e., 100%; for the medium structures, it drops to 0.3 or 30%; and for the very fine structures on the right side, it is only 5%. Under the condition that actually all structures, the coarse as well as the fine ones, should be imaged with the same contrast, the quality of the optics is better, the higher the contrast remains in relation to the number of line pairs per millimeter. The maximum resolution, in which we are causally interested, we determine where the finest structures are just still recognizable. MTF values between 5% and 2% are used to define „just visible“. In our case, this is 90 lp/mm.

MTF is only useful for determining the resolution of optics, silver films and digital image carriers because the output devices are in a range that can be easily evaluated by visual inspection or purely mathematical observation.

Next The resolving power of optics

Main Visual acuity

Previous Circle of confusion and diffraction slice – Not every aperture is a good aperture

If you found this post useful and want to support the continuation of my writing without intrusive advertising, please consider supporting. Your assistance goes towards helping make the content on this website even better. If you’d like to make a one-time ‘tip’ and buy me a coffee, I have a Ko-Fi page. Your support means a lot. Thank you!